Colloidal crystals: mimicking atomic behaviour
The earliest computer simulations dealt with the simplest
of shapes, such as hard-discs in 2d and hard-spheres in
3d. Even these basic particles can undergo phase
transitions, forming a crystalline phase upon
compression. Modern computers allow for increasingly more
complex shapes to be studied. Even slight particle
anisotropy is sufficient for a rich phase behaviour:
hard-sphere dumbbells, for example, which consist of two
rigidly fused hard spheres of equal size, can form liquid
phases, plastic crystalline phases (where the particle
centres of mass are aligned but they can rotate [relatively]
freely), aperiodic crystals (where the constituent spheres
of the dumbbells form a crystal, but the particle centres of
mass do not) and, finally, periodic crystals (where both the
constituent spheres and the centres of mass lie on lattice
sites).
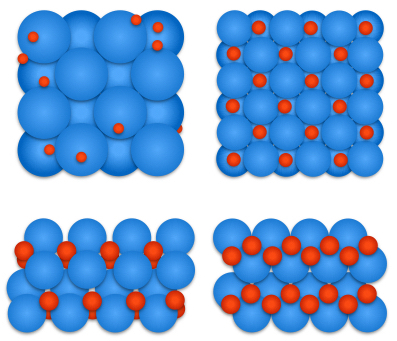
The phase behaviour becomes even richer if one considers
anisotropic dumbbells: two hard spheres of uneven size,
rigidly fused
together. Using
computer simulations, we have shown that such particles,
often named snowmen-shaped particles, can form a wide range
of stable crystalline phases depending on the ratio of the
diameters of the constituent spheres. Here the constituent
spheres form crystals with lattices that resemble such
atomic crystals as NaCl
(salt), CrB (chromium
boride), αIrV
and γCuTi. Another
interesting feature is that the phase behaviour of systems
of snowmen particles is richer than that of the
corresponding binary mixture of hard spheres. Binary hard
sphere mixtures will tend to only form the best packed
crystalline structure, while for snowman particles, there
are multiple ways of arranging the bonds connecting the two
spheres, giving rise to a degeneracy entropy that can
stabilize non-best packed structures.